Conceived by Peter Rasmussen & Wei Zhang
Coordinated by Stan Isaacs
Designed by Bill Darrah, Dec. 2003
Wood version by Josef Pelikan, March 2004
3D-printed, plastic version by George Miller, Feb. 2009
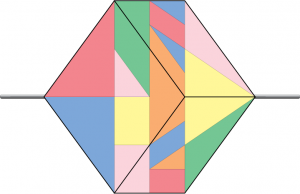
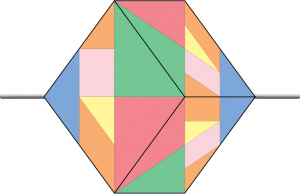
Tangram is a Chinese puzzle dating from the late eighteenth or early nineteenth century. It consists of seven flat pieces: two large triangles, two small triangles, a medium-size triangle, a square, and a parallelogram. These seven pieces can be arranged to form many shapes, including a square.

Conceiving of Tanacube
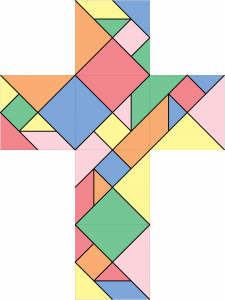
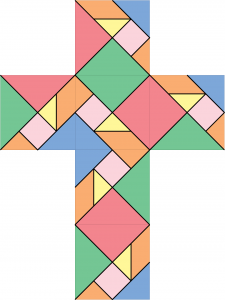
Our goal was to create a three-dimensional tangram. One idea was to create a set of three-dimensional pieces that could be used to build solid figures in much the same way that the seven flat tangram pieces are used to construct plane figures. However, we eventually settled on creating a set of three-dimensional puzzle pieces that could be put together to form what we called a “tangram cube”—a solid cube having six tangram faces. A bit of exploration determined that there are exactly two ways in which six identical tangram squares can be arranged to form the surface of a cube so that undivided edges join undivided edges and divided edges join divided edges, respectively.
These two arrangements result in two different cubes, Cube A, with what looks like a pair of large tetrahedra at opposite vertices separated by two parallel bands of tangrams, and Cube B, with what looks like a pair of small tetrahedra at opposite vertices separated by three parallel bands of tangrams.
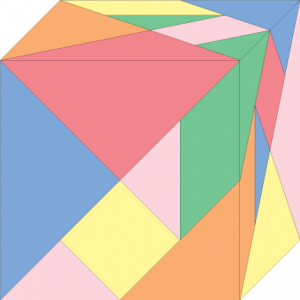
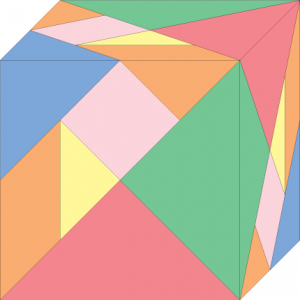
The tetrahedra and parallel bands of each cube are easiest to see if one pokes a skewer through the apexes of the two tetrahedra and then spins the cube on the skewer.
Basically, we had designed two versions of the outside of our new puzzle, but we realized that there were an infinite number of ways in which our tangram cubes could be dissected. So the problem became one of finding the dissection that would be the most interesting, challenging, and pleasing as a puzzle. We rejected the idea of a cube-packing assembly because of the need to produce an additional component—an open cube in which to pack the pieces—and the difficulty of rearranging pieces within such a cube. A free-standing assembly (like the Soma Cube) would have been satisfactory, but we agreed that an interlocking assembly that could be handled without falling apart would be best. Here are the criteria we settled on:
2. The cube has a “small” number of pieces;
3. Adjacent pieces are different colors;
4. The pieces interlock;
5. The cube’s edges are not split;
6. The cube has no void spaces;
7. There is a unique solution.
Building a Tanacube Model
Now it was time to build a model, and we chose to work with Cube A—the one with the pair of large tetrahedra. Our designer Bill Darrah first used Zome and Polydron construction sets to create large models of the pieces. Next he unfolded the Polydron pieces and traced their nets onto paper. We then made paper constructions of the pieces and revised them until we found the set we were looking for—six pairs of pieces, none of which are identical. Finally, the late Czech woodworker Josef Pelikan produced a beautiful wood version of the puzzle out of six varieties of hardwood, and we named it “Tanacube.”
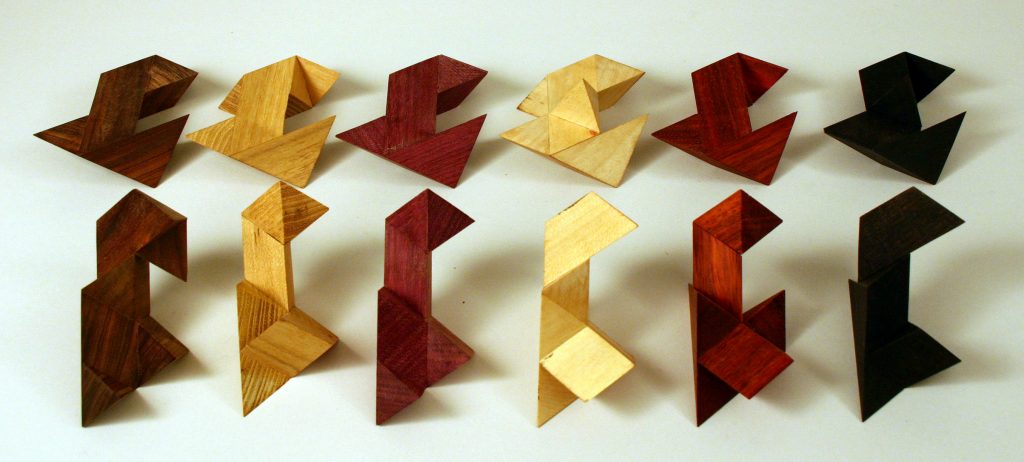
In February 2009 puzzle maker George Miller used his three-dimensional printer to publish a limited edition of a plastic Tanacube that has since become a coveted puzzle collector’s item.

Tanacube made by George Miller in 2009
Solving Tanacube
Tanacube has a unique solution in which adjacent pieces always have different colors. However the cube can also be assembled in such a way that some of the adjacent pieces have matching colors. It’s difficult enough to find one solution and a real challenge to find both.
[This story has an interesting side note. During the creation of Tanacube, George Miller told Dutch puzzle inventor Oskar van Deventer about our project. Oskar came up with his own unique and very different solution to the challenge. You can see Oskar demonstrating his Tanacube Too on YouTube.]
Adapted from the article “Tangram Cubes: A Collaborative Effort,” by Peter Rasmussen and Wei Zhang, published, in Cubism for Fun (no. 65, Nov. 2004), journal of the Nederlandse Kubus Club.